Keywords

Development Strategy, Small Organizations, Real Options.

JEL Classification: G32, L26, O21

Introduction

Modern corporations have ample opportunities to develop development strategies with an alternative choice of different options. For small businesses such opportunities are limited. According to Russian legislation, small business organizations include entities with a staff of up to 100 people, the volume of proceeds from the sale of goods (works, services) to 800 million roubles. This shows the significant economic potential of this type of business. However, a significant number of small organizations, according to statistics, function on the market from one to three years. This situation is due to the lack of sound strategic decisions, uncertainty and accompanied risks in production and economic activities.

The functioning of companies based on the financial strategy allows you to choose the appropriate areas of development in a changing environment. A tactical decision is recognized as rational if it is aimed at achieving the main strategic goal-improving the welfare of owners through maximizing the value of the organization. The use of the financial strategy allows ensuring the effective development of the organization, in which the mechanism of economic development of the organization is realized on the basis of mobilizing the internal potential and the possibility of manoeuvring financial resources. Strategic management of the organization can be considered as the management of its value. The advantage of the value model is the consideration of managerial flexibility. Management flexibility is based on the dynamics of development, which is expressed in taking into account possible changes in the strategy, depending on the transformation of the operating conditions.

Management flexibility in changing conditions, the presence of uncertainty is most adequately provided when using real options. A real option allows you to use business situations or implement an investment project to use changing situations to increase the value of your business. The effectiveness of the application of real options under conditions that are transformed is confirmed by the use in the activities of many companies, ensuring the flexibility of management decisions.

A study of developments on the application of real options in the development strategy of small businesses showed that at the present stage such problems are not completely resolved. In this article Torani, Rausser & Zilberman (2016) (develops a stochastic dynamic model of the adoption of solar PV in the residential and commercial sector under two sources of uncertainty-the price of electricity and cost of solar). In development Mun (2002) (real options are a useful tool to guide a firm's strategic planning and can create or enhance a firm's value). In development Trigeorgis & Reuer (2017) provides a review of Real Options Theory (ROV) in strategic management research, review the fundamentals of ROV and provide taxonomy of this research. In other studies (Kent Baker, Shantanu Dutta & Samir Saadi), it is noted that flexible management decisions in business practice are not widely used: Only 16.8% of enterprises in Canada use real options in the budgeting of capital. Research (Bartolomeu Fernandes, Jorge Cunha & Paula Ferreira) showed the effectiveness of using real options when justifying investments in the energy sector. A number of authors Mei & Clutter (2015) in this study, the real options approach are applied to analyse the timberland market. Integration of cooperation between large and small companies in the field of innovation is aimed at using real options in these processes, noted in other developments (David, Duane Ireland & Charles). In the work of Trigeorgis & Driouchi (2017) note that a contribution is made to the theory of multinationality and real options, considering the role of solid heterogeneity in realizing the real options for multinational corporations. The authors (Ahammad, Leone, Tarba, Glaister & Arslan, 2017) investigate the factors influencing the share of equity ownership sought in cross-border mergers and acquisitions (CBM&As). Drawing on real options theory and Transaction Cost Economics (TCE) they address and hypothesize key factors linked to commitment under exogenous uncertainty and the separation of desired and non-desired assets’ influence on share of equity sought by acquiring firms in CBM&As. In the study by Moschieri & Mair (2017), within the framework of corporate entrepreneurship, they quote "data on how partial alienation can be considered as real variants". Chen, Shen, Xue & Xia (2017) are investigating the (models the toll-adjustment mechanism as a real option to assess the value of flexibility of the right (but not obligation) to toll adjustments. Aretz & Pope (2017) reviews (Real Options Models of the Firm, Capacity Overhang and the Cross-Section of Stock Returns). Rau & Spinler (2017) note that (In light of low profitability and frequent alliance changes, the optimal choice of investment approach is addressed. This is achieved by comparing the performance of three investment approaches: Real options analysis and individual and collective discounted cash flow). Lawryshyn, Collan, Luukka & Fedrizzi (2017) note in their work (that the cash flow is used as an input in the continuous-time real option of valuation of each patent).

When investigating the applicability of real options in a small business, it is necessary to take into account the characteristics determined by the type of economic activity. The development strategy of small business organizations using the real options method allows taking into account risk situations in the activities of organizations.

The purpose of this study is to develop a methodology for determining the effectiveness of the application of real options (ROV) by small business organizations in conditions of uncertainty and risk.

The hypothesis is increasing the flexibility of the management decisions taken to justify the development strategy of small business organizations using the real options method.

Methods

Methods for making flexible management decisions based on real options are justified in high-risk situations. Real options for evaluating investments in the development of new drugs have been used by the pharmaceutical company Merck for many years. Another company Texaco, using an optional real method, estimated the oil fields in the initial stage of development, where the level of risks is much higher than at the stage of operation. Increased risks were accompanied by the evaluation and development of a strategy for the development of oil fields in the North Sea by British Petroleum. The method of real options was used to estimate the value of many companies.

The use of real options in Russian practice is not enough. In individual firms, the real option method is used in valuation: Pricewaterhouse Coopers Standard & Poor's, Baker Tilly, Rus Audit. At present, the process of using this method for choosing investment options begins in Russia.

In the areas of activity where changing factors negatively affect financial results, the method of real options is most in demand. Therefore, the management of companies chooses alternative ways to increase the value of business, while the flexibility of management decisions is seen as an asset that should be taken into account in business value.

The valuation method for real options is used mainly by large companies, in the small business there is no experience of applying this method. Although it is in small business, this method is in great demand. Small businesses are more exposed to risk situations than large corporations and need flexible financial decisions. For small businesses, it is especially important to take into account the positive factor of managerial flexibility, which will increase the attractiveness for investors. Reflect this factor in the evaluation can be based on the method of real options. The use of real options allows you to analyse the functioning of a small organization as a set of interrelated investment projects, which affects the increase in flexibility, the rationale for the goals.

Adopting flexible management decisions using real options affects the reduction of losses and the increase in the value of assets. Under conditions of uncertainty and changing operating conditions, the methodology for determining alternative strategic solutions is significant for the management of a small organization. The application of methods of real options is due to the following features:

1. The management of the firm can change management decisions with the appearance of new information influencing the investment evaluation;

2. The dependence of the asset value on the value of other assets;

3. The occurrence of certain conditions affects the cash flows generated by the asset under consideration;

4. The arrival of the latest information can change the uncertainty in making an investment decision.

The uncertainty of the options for the future development of a small organization determines the use of the real option method. The use of option methods does not provide accurate forecasts of development, but offers alternative options in the face of uncertainty and the presence of risks the concept of real options complements the theory of financial options and extends it to the sphere of economic analysis of investment efficiency in conditions of uncertainty and risk. With the help of ROV for non-financial assets, it is possible to overcome the shortcomings of traditional calculations using the reduced cost method (NPV). Based on the method of real options, it becomes possible to quantify the potentials existing in the project and include them in the evaluation of the effectiveness of the investment project. The net cash flow of an investment project using management options can be calculated as follows:

NPVexp ? NPVtr + ROV

Where: NPVexp (expanded NPV) -strategic net cash flow using real options; NPVtr (traditional NPV) -net cash flow calculated using the traditional method without the use of real options; ROV-Real Options Value.

When new information is received, the optional method allows you to change strategic plans to reduce losses and get the most revenue. With the use of real options, it is possible: Postponement of the adopted direction of development to the time of information change; reduction or suspension of negative processes that may occur in the implementation of the adopted direction of development; transformation of financial strategy in correspondence with changed situations; use of new development financing potentials and appropriate adjustment of capital structure. Preference for certain directions of the strategy is realized in accordance with the adopted development goals and therefore, the efficiency of functioning is increased.

Consider, for example, the calculation of the value of a real option based on the data of the small business organization XYZ. The organization expects to invest 150 million roubles to purchase a new production technology and in one year plans to receive an income from the use of this technology in the amount of 175 million roubles. In this situation, with a probability forecast of 50%, there are two options: Positive (income from use will grow to 200 million roubles). And negative (income from use will be reduced to 110 million roubles). A small organization can work for another year using the old technology, that is, it can postpone the acquisition of a new technology for one year. We accept the current discount rate of 8%. Based on this data, we calculate NPV (net present value):

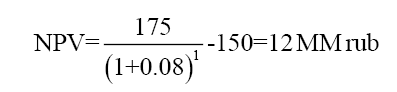
According to the requirements of investment analysis, the project is adopted with a positive NPV. The considered company XYZ accepts these investment conditions. We will calculate the options for a positive and negative investment of funds with an assumed probability of 50%.

Expected value of NPV for positive forecast:

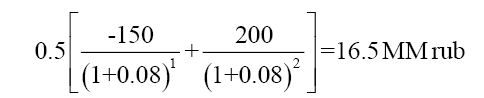
Expected NPV for negative forecast:

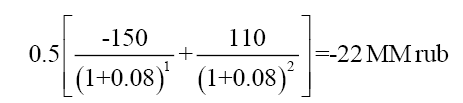
Calculations showed that after the first year, if the positive forecast is confirmed, the firm invests in a new technology, if not; XYZ will work on the old technology. If you delay the acquisition of a new technology for one year, the firm will receive an income of 16.5 million roubles. When investing in the current period, the company's income will be -12 million roubles. You can calculate the value of the option with the possibility of changing the investment conditions and without this change (16.5-12=4.5 million roubles). When using calculations using the discounted cash flow method, the current information is taken into account, when calculating an investment, the optional method takes into account changing information.

The present situation on the experience of applying real options in Russian practice is also due to the fact that there is no methodology for applying real options in various conditions. Analysis of various scientific sources on the study of the concept of real options reveals that in a significant part of the development there is not considered a specific methodology for using the option method.

Model

In the evaluation of financial options, the well-known Black-Scholes models, the Cox- Ross-Rubinstein model, the Merton model and others are used. The analysis of the models allowed distinguishing the key characteristics of real options: The possibility to use the option in a specific changing situation; the adoption of managerial decisions in accordance with a given goal; the possibility of accounting for uncertainty and risk. The Black-Scholes model allows you to calculate a real option based on two approaches:

For the European call option:

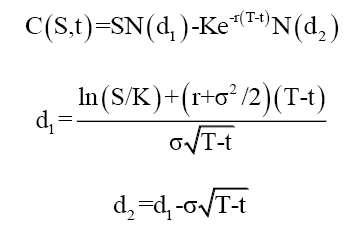
For the European put option:

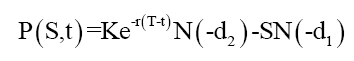
Where: C(S, t) -The current value of the call option at the moment t before the expiration of the option;

P(S, t) -The current value of put at the moment t before the expiration of the option;

S-Current price of the underlying asset;

N(x) -is the distribution function of the standard normal distribution;

K-is the exercise price of the option;

(r) -risk free interest rate;

(T-t) -is the time until the expiry of the option period (option period);

σ -yield volatility (the square root of the variance) of the underlying asset.

The Black-Scholes option evaluation model is applicable in cases where operations are performed continuously and in a very short time. The use of the Black-Scholes model to assess the market value of a real option under the conditions of the Russian economy is problematic, since the model includes the standard deviation of the asset's yield, which is difficult to estimate objectively. It should be noted that the scope of the Black-Scholes model is European options. By analogy with European options, the Black-Scholes model for making a managerial decision is executed in the future at a predetermined date. Greater volatility in the modification of prices for the underlying asset may distort the calculation using the Black-Scholes model. Therefore, in order to evaluate real options, in our opinion, a binomial model should be used.

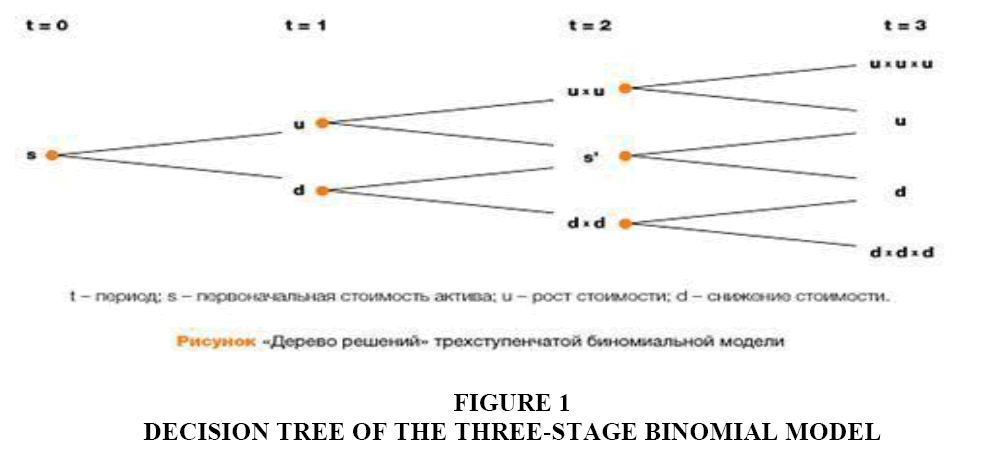
The basic idea of the binomial model includes modelling the movement of the value of the underlying asset using the binomial law. The model introduces the assumption of a change in the value of the underlying asset in two directions: With the probability "p" there is an increase in value or with a probability of "1-p", a decrease in value occurs. Thus, the stochastic modelling in time of the value of the underlying asset is carried out. With an increase in the number of considered periods of time, a binomial tree is obtained (Figure 1).

Calculations in the implementation of the binomial model are carried out in a number of stages. Initially, a lattice of an asset basis is constructed based on the product of the current value of the asset and the growth rate and the reduction criterion. At the lattice sites, the most appropriate solutions are determined and then they determine the effect that the chosen solutions can have on the results of the design in question. Next, an option valuation lattice is created based on the backward induction method. Then, the final nodes of the lattice are evaluated and the intermediate nodes are evaluated from right to left. The most suitable solution is selected at each lattice site. At this stage, you can determine the value of real options by subtracting the management solution from the calculated effect, taking into account the options of the basic effect without.

Using the binomial method, we introduce the assumption of discreteness and boundedness of the number of links. As a rule, the number of links corresponds to the frequency of making meaningful management decisions. The grid points reflect key moments in the decision-making process: Reduction/increase in production, the adoption of a new project, the abandonment of the project and others. If the business situation changes continuously, the time intervals between the nodes should be shortened and the number of links in the binomial lattice should be increased. In this case, the model under consideration is continuous, but not discrete, as in most cases.

According to the binominal pricing model, the Call option (or Put-does not matter) can take one of two values: Either U is the "maximum" or D is the "minimum" value. The calculation is made by the following formula:

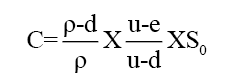
In the considered formula, the components are calculated as follows:

Where: S₀-is the exchange price of the asset on the date of the option agreement conclusion;

E-the price, at which the option is executed;

r-risk free annual interest rate of the financial market;

t-is the time from the purchase of the option to its execution (measured in years);

The price of an option is a certain fraction of the value of an asset on the date the contract is entered into. For an investor, there are only two probabilities:

1. The investor receives an income that is calculated as (u*S-X).

2. As a result of the transaction, the investor loses the funds invested in the purchase of the option (d*s-x).

The technique of constructing a binomial model is more cumbersome than the Black- Scholes method, but it allows obtaining more accurate results when there are several sources of uncertainty or a large number of decision-making dates, which is typical for small business organizations.

Calculation of the binomial model is possible in almost all programs for professional statistical analysis of data such as SPSS, SAS, R and others.

The binominal model thus formed allows predicting the dynamics of management decisions of small business organizations that correspond to specific values of the initial indicators.

Results and Discussion

The proposed procedure for making flexible management decisions using real options includes:

1. Analysis of the proposed project.

2. Identification of the type of risk.

3. Risk assessment.

4. Formation of a management strategy in a risk situation.

5. Embedding real options in decision making.

With the use of real options, it becomes possible not to strictly carry out the planned tasks, but, taking into account the changing conditions, to search for the most rational management decisions at the moment in time. Appropriate behavior is characterized by the adoption of managerial decisions in accordance with the theory of expected utility.

To evaluate the effectiveness of the chosen strategy on the basis of the binomial model, it is proposed to use the Bayes criterion for income generation. Let us analyse the criteria for choosing optimal strategies on the example of the small organization XYZ under consideration.

The choice will be made based on the use of the Bayes criterion (Table 1). The indicator B_i of the efficiency of the strategy S_i by the Bayes criterion is the mathematical expectation of the income of the itch line, taking into account the probabilities of all possible business conditions:

Table1 The Matrix of Optimal Strategy Selection
	T1	T2	T3	T4	T5	Bi
	P1=0.2	P2=0.1	P3=0.3	P4=0.1	P5=0.2
S1	150	170	200	210	160	160
S2	140	180	190	160	150	149
S3	210	160	180	200	150	162
S4	130	110	180	210	160	144
S5	170	180	200	180	130	156

The optimal among the pure strategies based on the Bayes criterion for wins is the strategy S_i* with the maximum average winnings

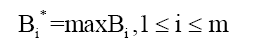
The exponent B_i is called the price of choice in pure strategies by the Bayes criterion.

According to the calculations, B_i* =162 million roubles-the price of choice in pure strategies by the Bayes criterion.

In substantiating the development strategy of small business organizations using the real options method, the following scheme is proposed:

1. The definition of critical control points in the binominal decision tree;

2. The situation of exercising the right to exercise the option;

3. Selection of real options most suitable for the management decision in question;

4. The way of modelling real options of the management decision;

5. Performance evaluation of the effectiveness of the use of the real options method for risk management based on the Bayes criterion.

The application of ROV helps to view the company's activities as a set of interrelated investment projects, which allows for greater flexibility and faster achievement of targets. To substantiate the strategy for small business development, it is recommended to use the following types of real options:

1. Option to choose the time of project implementation.

2. Option to suspend.

3. Option for a phased investment.

4. Option to reduce the scale of the project.

5. Option to change the final product.

6. Option to change resources.

The method of real options involves the construction of a scheme in which a chronological sequence of investment decisions is outlined and new opportunities are identified that need to be carefully examined before taking on the following investment-related commitments. Thus, the firm is able to determine the sequence of capital management decisions that will be made as the process develops, not at the beginning, when many key factors are still unknown.

Real options are used where the objective is to reduce risks, but not to receive high returns. The application of the theory of real options allows you to evaluate in monetary terms the company's opportunities and the dangers facing it. The main force of real options lies in their strategic application: They can become the fundamental basis of any company's development strategy, but especially important for small business organizations. In this case, there are a large number of calculation formulas and ready-made programs, in which it is sufficient to substitute parameter values and then the option price is calculated, which can be interpreted as the cost of the investment project and the strategy as a whole.

Conclusion

The use of real options allows justifying the development strategy of a small organization. In this case, the stochastic behavior of the value of an asset over time is simulated. The binomial approach assumes a phased implementation of calculations. Applying the binomial method to estimate the value of real options, the most rational decision in strategic development is chosen. Evaluation of the optimality of the adopted development strategy can be calculated on the basis of the Bayes criterion. The above examples of calculations demonstrate the simplicity and visibility of the proposed methods in the practice of small businesses. The advantage of the real option method is that this method can be used in all situations where there is uncertainty and risk. The traditional approach of performance assessment does not take into account changing business conditions, which is the main drawback of this method.

In the presence of changing factors of the external environment of the enterprise, the methodology for determining the directions of activity when an event occurs is of considerable value. It is from this point of view that the theory of value evaluation of options is important for the management of small business organizations. This is the practical significance of the method of real options, so that even loss-making projects can turn out to be profitable-both in terms of final calculations and in reality. Real options create competitive advantages for small businesses. An effective financial strategy in the face of limited financial resources reduces the risks of uncertainty. It gives organizations the opportunity to find and discover new opportunities that can distinguish them from other firms and create a competitive advantage. Real options can provide more differentiation mechanisms for firms operating under less favorable conditions.

Analysis of the conducted studies on the use of real options in conditions of uncertainty and risk has shown that there are no developments on the use of real options in the management of small business organizations. The proposed approach is authors from the point of view of the binominal model on the basis of the choice of a real option and evaluation of the optimality of the adopted strategy.

References

1. Ahammad, M.F., Leone, V., Tarba, S.Y., Glaister, K.W. & Arslan, A. (2017). Equity ownership in cross?border mergers and acquisitions by British firms: An analysis of real options and transaction cost factors. British Journal of Management, 28(2), 180-196.
2. Aretz, K. & Pope, P.F. (2017). Real options models of the firm, capacity overhang and the cross-section of stock returns. Journal of Finance, Forthcoming, 95.
3. Berk, A.S. & Podhraski, D. (2018). Superiority of Monte Carlo simulation in valuing real options within public-private partnerships. Risk Management, 20(1), 1-28.
4. Bernardo, A.E. & Chowdhry, B. (2002). Resources, real options and corporate strategy. Journal of Financial Economics, (2), 211-234.
5. Bowman, E.H. & Moskowitz, G.T. (2001). Real options analysis and strategic decision making. Organization Science, 12(6), 730-743.
6. Brealey, R.M. (2012). Principles of corporate finance (Second Edition). Moscow: ZAO Olympus-Business.
7. Bromiley, P., Rau, D. & Zhang, Y. (2017). Is R&D risky? Strategic Management Journal, 38(4), 876-891.
8. Carlsson, C. (2018). Digital coaching for real options support. Soft Computing Based Optimization and Decision Models, 153-175.
9. Ching-Ping, W., Hung-His, H. & Jin-Sheng, H. (2017). Reverse-engineering and real options-adjusted CAPM in the Taiwan stock market. Emerging Markets Finance and Trade, 53(3), 670-687.
10. Cong, L.W. (2017). Real options, business valuation and dynamic decisions. Journal of Management Policy and Practice, 18(3), 9-12.
11. Chalikias, M. & Skordoulis, M. (2017). Implementation of F.W. Lanchester's combat model in a supply chain in duopoly: The case of Coca-Cola and Pepsi in Greece. Operational Research: An International Journal, 17(3), 735-745.
12. Chalikias, M., Lalou, P. & Skordoulis, M. (2016). Modelling advertising expenditures using differential equations: The case of an oligopoly data set. International Journal of Applied Mathematics and Statistics, 55(2), 23-31.
13. Chalikias, M. & Skordoulis, M. (2014). Implementation of Richardson's arms race model in advertising expenditure of two competitive firms. Applied Mathematical Sciences, 8(81), 4013-4023.
14. Chen, Q., Shen, G., Xue, F. & Xia, B. (2018). Real options model of toll-adjustment mechanism in concession contracts of toll road projects. Journal of Management in Engineering, 34(1).
15. Damodaran, A. (2008). Investment valuation. Tools and techniques for valuing any assets. Moscow: Alpina Business Books.
16. Durand, R., Grant, R.M. & Madsen, T.L. (2017). The expanding domain of strategic management research and the quest for integration. Strategic Management Journal, 4-16.
17. Ioulianou, S., Trigeorgis, L. & Driouchi, T. (2017). Multinationality and firm value: The role of real options awareness. Journal of Corporate Finance, 46, 77-96.
18. Hagspiel, V., Hannevik, J., Lavrutich, M. & Naustdal, M. (2018). Real options under technological uncertainty: A case study of investment in a post-smolt facility in Norway. 158-166.
19. Kim, K., Park, H. & Kim, H. (2017). Real options analysis for renewable energy investment decisions in developing countries. Renewable and Sustainable Energy Reviews, 75, 918-926.
20. Lawryshyn, Y., Collan, M., Luukka, P. & Fedrizzi, M. (2017). New procedure for valuing patents under imprecise information with a consensual dynamics model and a real options framework. Expert Systems with Applications, 86, 155-164.
21. Lehmberg, D. & Davison, M. (2018). The impact of power distance and uncertainty avoidance on real options exercise: Potential for suboptimal time delays and value destruction. Journal of Behavioural Finance, 62-72.
22. Lund, D. & Nymoen, R. (2018). Comparative statics for real options on oil: What stylized facts? The Engineering Economist, 63, 54-65.
23. Mak, J., Cassidy, S. & Clarkson, P.J. (2017). Towards an assessment of resilience in telecom infrastructure projects using real options. Proceedings of the 21st International Conference on Engineering Design (ICED 17).
24. Mei, B. & Clutter, M.L. (2015). Evaluating timberland investment opportunities in the United States: A real options analysis. Forest Science, 61(2), 328-335.
25. Moschieri, C. & Mair, J. (2017). Corporate entrepreneurship: Partial divestitures as a real option. European Management Review, 14(1), 67-82.
26. Mun, J. (2002). Real options analysis: Tools and techniques for valuing strategic investments and decisions. John Wiley & Sons.
27. Nadarajah, S., Margot, F. & Secomand, N. (2017). Comparison of least squares Monte Carlo methods with applications to energy real options. European Journal of Operational Research, 256(1), 196-204.
28. Rau, P. & Spinler, S. (2017). Alliance formation in a cooperative container shipping game: Performance of a real options investment approach. Transportation Research Part E: Logistics and Transportation Review, 101(1), 155-175.
29. Rocha, K., Salles, L., Garcia, F. & Sardinha, J. (2007). Real estate and real options: A case study. Emerging Markets Review, 8, 67-79.
30. Rodrigues, S., Chen, X. & Morgado-Dias, F. (2017). Economic analysis of photovoltaic systems for the residential market under China's new regulation. Energy Policy, 101, 467-472.
31. Sabet, A.H. & Heaney, R. (2017). Real options and the value of oil and gas firms: An empirical analysis. Journal of Commodity Markets, 6, 50-65.
32. Tan, J.J. (2018). Interfaces for enterprise valuation from a real options lens. Strategic Change, 27(1), 69-80.
33. Torani, K., Rausser, G. & Zilberman, D. (2016). Innovation subsidies versus consumer subsidies: A real options analysis of solar energy. Energy Policy, 92, 255-269.
34. Trigeorgis, L. & Reuer, J.J. (2017). Real options theory in strategic management. Strategic Management Journal, 38(1), 42-63.
35. Triantis, A. & Borison, A. (2001). Real options: State of the practice. Journal of Applied Corporate Finance, 14(2), 8-24.